Investigation of Voltage Unbalance Problems In Electric Arc Furnace Operation Mode
Yacine DJEGHADER1 and Hocine LABAR2
1Department of Electrical Engineering,
2Department of Electrical Engineering,
E-mails: djeghaderyacine@yahoo.fr; labarhocine@yahoo.fr
*Corresponding author: Phone: 0021365741133
Abstract
In modern steel industry, Electric Arc Furnaces are widely used for iron and scarp melting. The operation of electric arc furnace causes many power quality problems such as harmonics, unbalanced voltage and flicker. The factors that affect Electric arc furnace operation are the melting or refining materials, melting stage, electrodes position (arc length), electrode arm control and short circuit power of the feeder, so, arc voltages, current and power are defined as a nonlinear function of arc length. This study focuses on investigation of unbalanced voltage due to Electrics Arc Furnace operation mode. The simulation results show the major problem of unbalanced voltage affecting secondary of furnace transformer is caused by the different continues movement of electrodes.
Keywords
Electric Arc Furnace ELE; Modelling; Power Quality; Unbalanced Voltage.
Introduction
The first electric arc furnaces were developed by Paul Héroult of France, with a commercial plant established in the United States in 1907. Initially "electric steel" was a specialty product for such uses as machine tools and spring steel [1-3].
The precise control of chemistry and temperature encouraged use of electric arc furnaces during World War II for production of steel for shell casings. Today steelmaking arc furnaces produce many grades of steel, from concrete reinforcing bars and common merchant-quality standard channels, bars, and flats to special bar quality grades used for the automotive and oil industry. The steelmaking arc furnace is generally charged with scrap steel, though if hot metal from a blast furnace or direct reduced iron is available economically, these can also be used for steelmaking [2]. Because of the very dynamic quality of the arc furnace load, power systems may require technical measures to maintain the quality of power for other customers; flicker, unbalanced voltage and harmonic distortion are common effects of arc furnace operation on a power system [4].
The Electric Arc Furnace, designed for steelmaking from recycled scrap iron (Figure 1) :
§ Furnace charging: the scrap and the additives (lime, coal…) are loaded into special charging buckets which are then emptied into the furnace;
§ Melting: an electric arc is created between the graphite electrodes and the scrap which entails the charge melting and the formation of a steel bath covered by a slag layer, volatile solute species (e.g. zinc) begin to be removed;
§ Refining: in this step of the process, phosphorus is removed from the steel bath by interfacial reactions between the slag and the liquid metal, injection of oxygen promotes the decarburization reaction with dissolved carbon and bubbles of carbon monoxide (CO) are formed, which helps to remove other dissolved gases;
§ Slag foaming: the CO-bubbles crossing the slag layer make it foam, the foaming process being enhanced by the addition of coal powder;
§ Casting: after the composition and the temperature of the bath have been controlled, the liquid steel is cast [5].
The instability and non-linearity are greatest during melting down of cold scrap. The delay and erratic process of striking the arc and resulting gaps in the current are conspicuous. As melting down progresses, the striking becomes more stable, but the current can still contain low-frequency fluctuations. The temperature and heat of the arc are high with a liquid steel bath, and the thermal conduction is low [6].

Figure 1. Typical electrical arc furnace
The EAFs are time-variant and non-linear loads and create the power quality problems such as unbalanced voltages and currents, voltage fickers as well as odd and ven harmonics. These problems need to be rectifed in the EAF. In this work we focused on the problems of unbalanced voltage in electric arc furnace operation.
Model Description
Our Electric arc furnace melt steel, by applying an AC current to a steel scrap charge by means of graphite electrodes. It requires about 520 kwh/ton, and produce 700t/year approximately (figure 2).
All the processes of electrical arc furnace can be summarized in figure 3 [4-9]. We have record 32 measurements of each measured parameter for 9 transformer taps. Normal operation must make the compromise between the limitations according to maximum power (Smax) and acceptable current (Ie) respectively [An,1 An,2] and [Bn,1 Bn,2], where “n” is tap index. Then conferring itself to this constraint the adjustment law of electrodes position will be done according to maximum power.
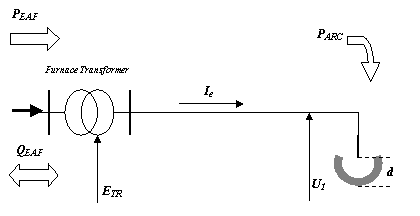
Figure 2. Studied Model
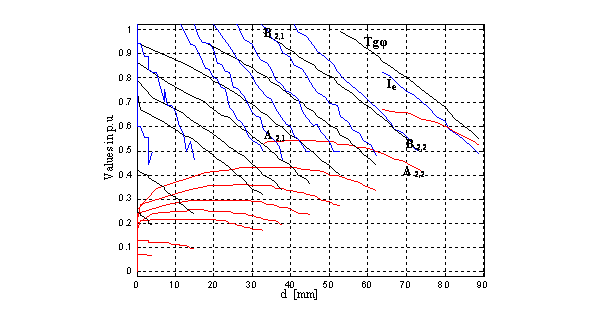
Figure 3. Electric Arc Furnace Process
Modelling of Alternating Current Electric Arc Furnace
The Electric arc furnace is modelled together with the neighbouring network (fiure 2). The circuit equation of the furnace transformer (Etr), to the end of electrodes can be writhen as follow:
Etr = √3∙Z1∙Ie+U1 (1)
where U1, Ie and Z1 are respectively electrode voltage, current and impedance of electric arc furnace transformer with flexible cable
Then,
![]() (2)
(2)
![]() (3)
(3)
![]() (4)
(4)
 (5)
(5)
where: R1 is the resistance of electric arc furnace transformer with flexible cable; X1 is the reactance of electric arc furnace transformer with flexible cable; PEAF is total active power of the furnace; Parc is the active power of arc.
So, from equations (2,3,4) we can deduct
![]() (6)
(6)
and
QEAF = Qarc + ΔQ (7)
![]() (8)
(8)
where: QEAF is total reactive power of electric arc furnace; Qarc is the reactive power of arc;
![]() (9)
(9)
where: Rarc and Xarc : are respectively the resistance and reactance of electric arc.
Following to the treatment, an empirical model is proposed [11]:
Rarc = AR(u)eα(u)d
where: ![]() ,
,
![]() , Xarc=AX(u)d2
+ BX(u), AX = 1.05∙10-2∙e0.075(90-U),
BX = 3.14/153 – 3.10-2e0.075(90-U), d is the
distance between electrode and scrap.
, Xarc=AX(u)d2
+ BX(u), AX = 1.05∙10-2∙e0.075(90-U),
BX = 3.14/153 – 3.10-2e0.075(90-U), d is the
distance between electrode and scrap.
Zarc = Rarc(U,d) + jXarc(U,d) (10)
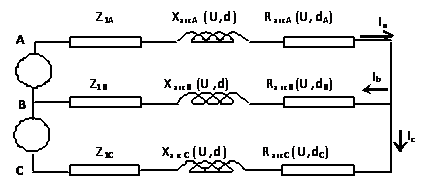
Figure.4 Equivalent circuit of the impedance of Electric arc furnace
The equations of the stitches:
Uab = Ia(2Z1 + ZarcA + ZarcB) – Ic(Z1 + ZarcB)
Ubc = -Ia(Z1 + ZarcB) + Ic(2Z1 + ZarcA + ZarcB) (11)
Ib = Ia + Ic
The matrix shape is as follows:
[U] = [Z].[I]
[I] = inv(Z).[U] (12)
The arcs voltages calculated according to the following equations:
UarcA = ZarcA ∙ Ia
UarcB = ZarcB ∙ Ib (13)
UarcC = ZarcC ∙ Ic
Components, we just replace the vector U by I in expression (13).
Problem of Unbalanced Voltage
In a balanced sinusoidal supply system the three line-neutral voltages are equal in magnitude and are phase displaced from each other by 120 degrees (Fig. 6a). Any differences that exist in the three voltage magnitudes and/or a shift in the phase separation from 120 degrees is said to give rise to an unbalanced supply (Fig. 6b) [12].

Figure 5. Electric Arc Furnace Operation Mode
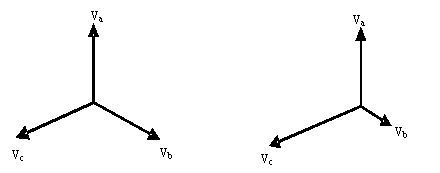
(a) (b)
Figure 6. Balanced & An Unbalanced system
Causes of voltage unbalance include unequal impedances of three-phase transmission and distribution system lines, large and/or unequal distribution of single-phase loads, phase to phase loads and unbalanced three-phase loads. When a balanced three-phase load is connected to an unbalanced supply system the currents drawn by the load also become unbalanced. While it is difficult or virtually impossible to provide a perfectly balanced supply system to a customer every attempt has to be taken to minimise the voltage unbalance to reduce its effects on customer loads [13].
Unbalanced Voltage Quantification
To quantify a voltage or current unbalance for a three-phase system, the so-called Fortescue components or symmetrical components are used. The three-phase system is decomposed into a so-called direct/positive-sequence, inverse/negative-sequence and homopolar/ zero-sequence system [8].
- d = direct
- i = invers
- o = homopolar
once calculated using matrix transformations of the three-phase voltage or current phasors. The expressions below (13) are formulated for the voltage “U”, if one need to define the current
 (14)
(14)
where the rotation operator “a” is given by: a = e-j120
These transformations are energy invariant, so any power quantity calculated with the original or transformed values will result in the same value [12-13].
The inverse transformation is:
 (15)
(15)

Figure 7. Symmetrical components of an unbalanced system of voltages
The ratios kU (voltage) and kI (current) between the magnitudes of negative and positive sequence components of voltage and current respectively are a measure of the unbalance (in %):
Ku = Ui/Ud∙100% (16)
Application
Four tests are made corresponding to the different transformer taps (190, 210, 234 and 265 V) with uncertainty of 20 % in the distances dA and dC.
The figures represent the three arc voltages UarcA, UarcB, UarcC, with Symmetrical components for unbalanced voltage, and. homopolar (Uo), direct (Ud) and invers(Ui).
The Simulation results are represented in figure 8.
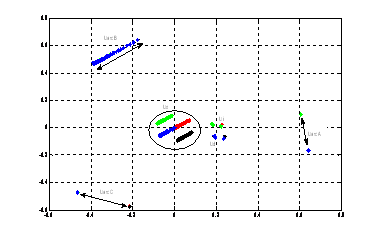
Figure 8. Simulations results: (a) Voltage arc Electrode « 190V »
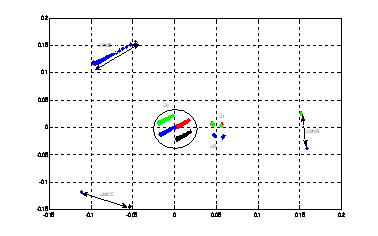
Figure 8. Simulations results: (b) Voltage arc Electrode « 210V »
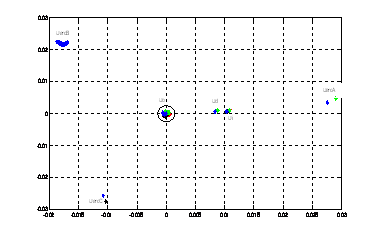
Figure 8. Simulations results: (c) Voltage arc Electrode « 234V »
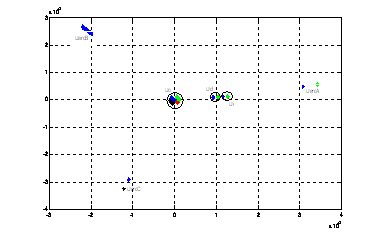
Figure 8. Simulations results: (d) Voltage arc Electrode « 265V »
Our simulation gives the results represented in table 1.
Table 1. Testing results
|
|
190V |
210V |
234V |
265V |
|
kU(%) |
4.0566 |
4.0530 |
4.0122 |
3.9940 |
|
kI(%) |
4.0222 |
4.0491 |
4.0171 |
4.0226 |
International standards
(e.g. EN-50160 or the IEC 1000-3-x series) give limits for the unbalance ratio
defined by (16) of < 2 % for
Conclusions
Voltage and current unbalance problems of power networks caused by AC EAF operation are studied with a new closely parametrical model taking in account electrodes positions and transformer taps.
The simulation results show that the homopolar/zero-sequence displacement takes one position in each stage in EAF operation mode , but the other arcs voltages (UarcA and UarcC) take two positions corresponding to dmax (electrode in high positions) and dmin (electrode low position) for all test stages, the same deduction can be obseved for direct and inverse. So homopolar compenent obey to hysterisis phenomenum.
This study has shown an important voltage and current unbalance in EAF operation when the ratios kU (voltage) and kI (current) increase over 2% (International standards requirements).
Annex. EAF characteristics
|
EAF type |
80LHF12,5 |
|
Transformer rating |
12.5 [MVA] |
|
Short circuit reactance |
2.9 [m?] |
|
Maximum electrode current |
30.84 [kA] |
|
Number of voltage taps |
9 |
|
Voltage range |
[90V÷265V] |
|
Primary voltage |
63 [kV] |
|
Weight capacity |
80 t |
|
Temperature gradient |
3 ÷ 4 °C/mn |
|
Furnace diameter |
2.47 [m] |
|
Electrode diameter |
0.35 [m] |
|
Distance electrode to wall |
0.71 [m] |
References
1.
Fenghua W., Zhijian J.,
Application of Extended Kal-man Filter to the modelling of Electric Arc
Furnace for Power Quality Issues,
2.
Carpinelli G., Iacovone
F., Russo A., Varilone P., Chaos-based modelling of DC arc furnaces for
power quality issues,
3.
Mokhtari H., Hejri M., A
New Three Phase Time-Domain Model for Electric Arc Furnaces Using MA
4.
Ma T., Strattonn B., Control
System and Method for Voltage Stabilization in Electric Power
5.
Zheng T., Makram E.B., An
Adaptive Arc Furnace Model,
6. Ma T., Sedighy M., Perkins B., Gerritsen T., Rajda J., Power Control System for AC Electric Arc Furnace, United States Patent 6,603,795 B2, August 5, 2003.
7. Surapong C., Yu C.Y., Thukaram D., Damrong K., Minization of the Effects of Harmonics and Voltage Dip Caused by Electric Arc Furnace, IEEE Conference 2000, pp. 2568-2576.
8.
Annette V.J., Basudeb
B., Assessment of voltage unbalance,
9. Timm K., Circle diagram of AC-Furnaces, Electrical Engineering of Arc Furnaces Symposium, Kehl, Germany, 18-21 April 2005.
10. Schau
H., Stade D., Mathematical modeling of three-phase arc furnaces. In
Proceedings of the IEEE-IC
11. Djeghader Y., Labar H., Bounaya K., Analysis of Harmonics Generated by Different Structures of a DC EAF, International Review on Modelling and Simulations - October 2008, pp. 173-177.
12. Chen S.L., Li R.J., P.H. His, Traction system unbalance problem, Analysis methodologies, IEEE Trans. on Power Delivery, 2004, 19(4), p. 1877-1883.
13. Awad H., Svensson J., Bollen M., Mitigation of Unbalanced Voltage Dips Using Static Series Compensator, IEEE Trans. On Power Elec., 2004, 19(13), p. 873-846.