Dependence of Ionicity and Thermal Expansion Coefficient on Valence Electron Density in A^IIB^IVC₂^V Chalcopyrite Semiconductors

Amar BAHADUR^1* and Madhukar MISHRA²

¹ Department of Physics, Kamla Nehru Institute of Physical and Social Sciences, Sultanpur- 228 118 (U.P.), India.

² Department of Physics, Birla Institute of Technology and Science, Pilani - 333 031 (Rajasthan), India

E-mail(s): (1) abr.phys@gmail.com; (2) madhukar@bits-pilani.ac.in

^* Corresponding author: Phone: +91- 9451431428

Abstract

A striking correlation has been found to exist between the free electron density parameter, average bond length, homoplar energy gap, heteropolar energy gap, ionicity and thermal expansion coefficient for A^IIB^IVC₂^V chalcopyrite semiconductors. The estimated values of these parameters are in good agreement with the available experimental values and theoretical findings. The electron density parameter data is the only one input data to estimate all above properties.

Keywords

Electron density parameter; Bond ionicity; Thermal expansion coefficient; Ternary chalcopyrite semiconductors.

Introduction

In recent years, ternary chalcopyrite semiconductors have attracted considerable attention because of their potential applications in the field of light emitting diodes, non-linear optics, photovoltaic devices and solar cells [1-4]. The solid solutions of these semiconductors have been used in electro-optic devices [5-7]. Their mixed crystals are being used for fabrication of detectors, lasers and integrated optic devices such as switches, modulators and filters etc. These chalcopyrites have many other practical applications in the areas of fiber optics, sensors and communication devices. In spite of their promising applications, some physical properties of these compounds have still not been significantly investigated. Frequent attempts have been made at understanding the crystal ionicity of these compounds. Phillips [8], Van Vechten [9-10], Levine [11] and other researchers [12-13] have developed various theories and calculated crystal ionicity for the case of simple compounds. Phillips and Van Vechten have calculated the homopolar and heteropolar contribution to the chemical bond in the binary crystals. Chemla [14] and several workers [15-16] have extended this theory to some complex crystals, neglecting the effect of noble metal d electrons. Levine [17] has extended Phillips and Van Vechten (PVV) theory of bond ionicity in case of various types of complex compounds considering the effect of d core electrons. It is clear form the Levine’s modifications and as well as PVV theory that the homopolar energy gap depends upon the nearest neighbor distance, while heteropolar energy gap is a function of nearest neighbor distance and the number of valence electron taking part in bond formation. Kumar et al. [18-19] have calculated these parameters in terms of plasmon energy because plasmon energy depends directly on the effective number of valence electrons in a compound. In all the above investigations, the ionicity has been evaluated in terms of nearest neighbor distance, valence and plasmon energy.

In recent years, various electronic and mechanical properties of ternary tetrahedral semiconductors have been explained using plasma oscillation theory [20-22], which leads to the fact that these physical properties depend directly on effective number of valance electrons and the density of the conduction electrons. The plasmon energy is related to the effective number of valence electrons as,

                                                                                                      (1)

whereis the effective number of valence electrons,  is the charge, is the mass of electron andis the plasmon energy is given by relation [23] as:

                                                                                                 (2)

where, is effective number of electrons taking part in plasma oscillation,  is the density and is the molecular weight of compound. This equation is valid for free electron model but to a fairly good approximation it can also be used for semiconductors and insulators. The valence electron density is described by electron density parameter which also depends upon the density of the conduction electrons and effective number of valence electrons according to relation [24],

                                                                                                    (3)

We, therefore, explore in the current work a new method for correlating electron density parameter with the crystal ionicity and thermal expansion coefficient for ternary chalcopyrite semiconductors. In the proposed approach, electron density parameter is the only input required for computation of the average bond length, homopolar energy gap, heteropolar energy gap, ionicity and thermal expansion coefficient.

Theory and Calculation

Average Bond Length, Homopolar Energy Gap, Heteropolar Energy Gap And Ionicity

The average bond length (d) is also related to the effective number of valence electrons, so there must be some correlation between bond length and electron density parameter. Using eqn. (1) and (3), we can express number of free electrons in terms of r_s as-

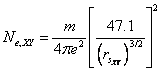(4)

In tetrahedral semiconductors, the effective number of valence electrons N_e,XY can also be expressed in terms of individual bond properties as-

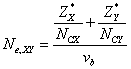                                                                                                     (5)

where, and are the number of valence electrons of the atom X and Y respectively, in XY compounds. The N_CX , N_CY  and N_CZ are coordination number of atoms and is bond volume. For A^IIB^IVC₂^Vsemiconductors, N_CA = N_CB = N_CC = 4, , and . Thus for A-C and B-C bonds, eqn. (5) becomes-and , respectively. With the help of these values and eqn. (4), we get following simple correlation between average bond length and free electron density parameter forand bonds as-

                                                                                                        (6)

                                                                                                        (7)

The average energy gapcan be separated into the homopolar and heteropolar parts according to following relation:

                                                                                                (8)

which yield following relation for bond ionicity (f_i):

                                                                                                (9)

where, ,are homopolar energy gap, ionic gap, respectively, given by relations as:

                                                                                                              (10)

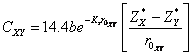                                                                                      (11)

where d_XY is distance between atoms X and Y, K_s is Thomas- Fermi momentum, , is Thomas-Fermi screening factor which is related to the effective number of free electrons in compound and b is prescreening constant.

With the help of eqn. (6) - (7) and (10), homopolar part of energy of individual bonds can be expressed in terms of free electron density parameter as:

                                                                                                    (12)

                                                                                                    (13)

The physical meaning of Eq. (11) is that  is given by the difference between the screened Coulomb potentials of atoms and having core charges  and . These potentials are to be evaluated at the covalent radii . Only a small part of the electrons are in the bond, the rest screen the ion cores, reducing their charge by the Thomas- Fermi screening factor , which affects the chemical trend in a compound. This screening factor, as well as the bond length, is related to the effective number of free electrons in a compound. Also, the electron density parameter directly depends upon the effective number of valence electrons. Thus, there must be some correlation between the physical processes which involve the ionic contribution  to the average energy gap  and the electron density parameter .

The expression for Thomas- Fermi momentum is given by relation as:

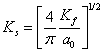                                                                                                            (14)

where, is Bohr radius (0.529) and  , the Fermi wave vector given by relation as: . Using eqn. (4), the Fermi wave vector can be expressed in terms of   as: and accordingly Fermi- momentum becomes-

                        (15)

Using the values of Eq. (15), the heteropolar energy can be expressed in terms of as-

                                                                                  (16)

here, , is 3 and 1, for  and bond, respectively, in A^IIB^IVC₂^V semiconductors. The avergae value of prescreening constant (b) has been taken as 1.3966 (Ref. 17) and 2.4516 (Ref. 17) for  and  bond, respectively. Using these values, we obtain expressions for heteropolar energy gap for  and  bond as:

                                                                                           (17)

                                                                                           (18)

The ionicity of  and  bonds in A^IIB^IVC₂^V semiconductors have been calculated using Eq. (9). The ionicity of both bonds ( and ) exhibit a linear relationship when plotted against electron density parameter. In fig. 1, we observe that in plot of ionicty and electron density parameter, the  bond lie on line nearly parallel to the  bond and the ionicity trends in these bonds increases with increasing electron density parameter. Based on above discussion, we propose the following equation for the calcualation of the ionicity of ternary chalcopyrite semiconductors,

                                                                                                                   (19)

where andare constants, The numerical values of these constants for  and  bonds are 0.4014 and 0.1651 and 0.265 and 0.365 respectively.

Table 1. Properties of A^IIC^V bond in A^IIB^IVC₂^V compounds

Compounds	Electron density parameter	Average bond length	Homopolar energy gap	Heteropolar energy gap	Ionicity
					Present work	Levine [17]
ZnSiP2	2.12	2.38	4.653	4.557	0.4895	0.438
ZnGeP2	2.13	2.39	4.605	4.515	0.4902	0.442
ZnSnP2	2.15	2.42	4.459	4.388	0.4920	0.455
ZnSiAs2	2.20	2.46	4.245	4.200	0.4948	0.436
ZnGeAs2	2.21	2.48	4.168	4.133	0.4957	0.422
ZnSnAs2	2.24	2.51	4.061	4.038	0.4971	0.450
CdSiP2	2.28	2.56	3.854	3.852	0.4998	0.539
CdGeP2	2.28	2.56	3.862	3.859	0.4997	0.532
CdSnP2	2.31	2.59	3.755	3.762	0.5011	0.536
CdSiAs2	2.35	2.64	3.572	3.596	0.5034	0.553
CdGeAs2	2.35	2.64	3.565	3.590	0.5035	0.549
CdSnAs2	2.38	2.67	3.473	3.505	0.5047	0.553

Table 2. Properties of B^IVC^V bond in A^IIB^IVC₂^V compounds

Compounds	Electron density parameter	Average bond length	Homopolar energy gap	Heteropolar energy gap	Ionicity
					Present work	Levine [17]
ZnSiP2	1.85	2.25	5.304	2.705	0.2064	0.177
ZnGeP2	1.90	2.32	4.926	2.532	0.2090	0.219
ZnSnP2	2.04	2.49	4.156	2.171	0.2143	0.298
ZnSiAs2	1.93	2.35	4.779	2.464	0.2100	0.220
ZnGeAs2	1.97	2.41	4.501	2.334	0.2119	0.182
ZnSnAs2	2.10	2.56	3.870	2.033	0.2163	0.135
CdSiP2	1.84	2.25	5.346	2.724	0.2061	0.191
CdGeP2	1.91	2.33	4.909	2.524	0.2091	0.231
CdSnP2	2.04	2.49	4.152	2.169	0.2144	0.298
CdSiAs2	1.92	2.35	4.789	2.469	0.2099	0.234
CdGeAs2	1.98	2.42	4.436	2.304	0.2124	0.199
CdSnAs2	2.11	2.57	3.813	2.006	0.2167	0.148

Generalizing Eqs. (6)-(7), (12)-(13), (17), (18) and (19), the expression for average bond length, homoploar, heteropolar energy gap and ionicity can be written as:

                                                                                                                 (20)

                                                                                                              (21)

                                                                                                   (22)

                                                                                                                 (23)

where and  are constants depending upon bonds of compound. Form these Eqs., it follows that bond properties can be evaluated form free electron density parameter. The free electron density parameter has been calculated from eqn. (3). Using Eq. (6)-(7), (12)-(13), (17), (18) and (19), we have calculated the average bond length, homopolar gap, heteropolar energy gap and bond ionicity for  and  bond in A^IIB^IVC₂^V semiconductors and presented in Table-1 and 2, respectively. For comparison, other estimates of these parameters are also presented. In most of compounds, our calculated values are in good agreement with the earlier reported values [17].

Figure 1. Plot of ionicity (f_i) and electron density parameter (r_s) for A^IIB^IVC₂^V chalcopyrite semiconductors. ● corresponds to the II-V bond and ♦ corresponds to IV-V bond in A^IIB^IVC₂^V chalcopyrite semiconductors. In this figure all data are taken from calculated values, which are presented in Table 1 and 2

Thermal Expansion Coefficient

Based on thermal expansion coefficient data, Neumann [25] has proposed following expression for the average thermal expansion coefficient for binary tetrahedral semiconductors:

                                                                                                   (24)

where  A is constant, T_m  is melting temperature, d is the bond length. The value of A is 0.021 for all tetrahedrally coordinated compounds (A^IV, A^IIIB^V, A^IIB^VI) as estimated from a hard sphere model based on the diamond structure. The value  is equal to the bond length of diamond i.e.. The ternary chalcopyrites of general composition A^IIB^IVC₂^V can be considered as similar to those of A^IIB^IV and A^IIB^VI. Thus, Eq. (24) can also be reasonably used to describe the thermal expansion coefficient of the ternary chalcopyrite. From Eq. (6) and (7), we get following relation betweenandas:

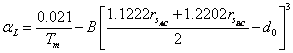                                                            (25)

For A^IIB^IVC₂^V chalcopyrite semiconductors, the values of B andare 16.1 () and 1.573, respectively [25]. Using Eq. (25), we have calculated the thermal expansion coefficient for A^IIB^IVC₂^V, ternary chalcopyrite semiconductors and presented in Table 3 along with experimental data and theoretical findings.

Table 3. Thermal expansion coefficient of A^IIB^IVC₂^V compounds

Compounds	Tm(K) [25-26]	αL = (10^-6K^-1)
		Present work Eq. (25)	Ref. [25]	Exp. [25, 27]
ZnSiP2	1643	6.15		6.3
ZnGeP2	1298	8.52		6.7
ZnSnP2	1203	6.57		3.2
ZnSiAs2	1369	5.99	5.3
ZnGeAs2	1148	7.62	6.7	1.0
ZnSnAs2	1048	5.80	4.5	2.3
CdSiP2	1393	5.83	7.0	7.2
CdGeP2	1073	9.00		6.1
CdSnP2	843	10.5	4.9
CdSiAs2	1120	6.16	5.0
CdGeAs2	938	8.19		6.0
CdSnAs2	871	5.56		4.7

Summary and Conclusion

The present relation may be considered to be first attempt to obtain simple correlations between free electron density parameter, average bond length, homopolar energy gap, heteropolar energy gap, ionicity and thermal expansion coefficient for ternary chalcopyrite semiconductors. These equations can be considered advantageous over others in the sense that it relates the electronic and thermal properties of semiconducting compounds with the free electron density parameter. The proposed relations yield not only satisfactory results, but also a comparison with the standard data provides a direct and precise check of the validity. Therefore, it is possible to predict the order of homopolar and heteropolar energy gap and the ionicity of semiconducting compounds from their free electron density parameter. The reasonable agreement between our calculated and previously known values of α_L indicates that the proposed relations are both useful and accurate for estimating thermal properties for binary tetrahedral semiconductors. Hence, we conclude from present analysis that the ionicity and thermal properties in semiconducting compounds can be evaluated from density of valence electrons. In the proposed approach, the calculation is simple, fast and more accurate. The only information needed is electron density parameter; no other experimental values are required. It is natural to say that present approach can easily be extended to the other more complex crystals.

Acknowledgement

We are thankful to Dr. A.K. Srivastava (H.O.D., Department of Physics and Electronics, K.N.I.P.S.S., Sultanpur, U.P., India) for his continuous inspiration and helpful discussions.

References

1.      Shay J. L., Wernick J. H., Ternary Chalcopyrite Semiconductors: Growth, Electronic Properties and Applications, New York, Pergmon Press, 1975.

2.      Vurgaftman I., Meyer J. R., Ram-Mohan L. R., Band parameters for III–V compound semiconductors and their alloys, Jour. Appl. Phys., 2001, 89, p. 5815-5875.

3.      Medvedkin G. A., Voevdin V. G., Magnetic and optical phenomena in nonlinear optical crystals ZnGeP₂ and CdGeP₂, Jour. Opt. Soc. Am. B, 2005, 22, p. 1884-1898.

4.        Jiang X., Lambrecht W. R. L., Electronic band structure of ordered vacancy defect chalcopyrite compounds with formula II-III₂-VI₄, Phys. Rev. B, 2004, 69, p. 035201-035209.

5.      Narain S., Analysis of the Debye Temperature for A^NB^8–N Type Ionic and Partially Covalent Crystals, Phys. Stat. Solidi (b), 1994, 182, p. 273-278.

6.      Neumann H., Simple theoretical estimate of surface energy, bulk modulus, and atomization energy of A^IB^IIIC^VI compounds, Cryst. Res. Tech., 1983, 18, p. 665-670.

7.      Moss T. S., Relations between the Refractive Index and Energy Gap of Semiconductors, Phys. Stat. Solidi (b), 1985, 131, p. 415-427.

8.      Phillips J. C., Dielectric Definition of Electronegativity, Phys. Rev. Lett., 1968, 20, 550-553; Ionicity of the Chemical Bond in Crystals, Rev. Mod. Phys., 1970, 42, p. 317-356.

9.      Phillips J. C., Van Vechten J. A., Spectroscopic Analysis of Cohesive Energies and Heat of Formation of Tetrahedrally Coordinated Semiconductors, Phys. Rev. B, 1970, 2, p. 2147-2160; Nonlinear Optical Susceptibilities of Covalent Crystals, Phys. Rev., 1969, 183, p. 709-711.

10.    Van Vechten J. A., Quantum Dielectric Theory of Electronegativity in Covalent Systems. I. Electronic Dielectric Constant, Phys. Rev., 1969, 182, p. 891-905.

11.    Levine B. F., d-Electron Effects on Bond Susceptibilities and Ionicities, Phys. Rev. B, 1973, 7, p. 2591-2600.

12.    Penn D. R., Wave-Number-Dependent Dielectric Function of Semiconductors, Phys. Rev., 1962, 128, p. 2093-2097.

13.    Singh O. P., Gupta V. P., Comments on Levin's Modifications in the Phillips and Van Vechten Theory. d- Electron Effect on Ionicity, Phys. Stat. Solidi (b), 1986, 137, p. 97-101.

14.    Chemla D. S., Dielectric Theory of Tetrahedral Solids: Application to Ternary Compounds with Chalcopyrite Structure, Phys. Rev. Lett., 1971, 26, p. 1441-1444.

15.    Fischer J. E., Glickman M., Van Vechten J. A., Proceedings of the International Conference on the Physics of Semiconductors, Roma, Italy, 1976.

16.    Neumann H., About the average bond ionicity in A^IB^IIIC^VI compounds, Cryst. Res. Tech., 1983, 18, p. 1391-1396.

17.    Levine B. F., Bond susceptibilities and ionicities in complex crystal structures, J. Chem. Phys., 1973, 59, p. 1463-1487.

18.    Kumar V., Prasad G. M., Electronic properties of ionic rocksalt crystals, Jour. Phys. Chem. Solids, 1989, 50, p. 899-901.

19.    Srivastava V .K., Homopolar and heteropolar energy gaps in zincblende crystals, J. Phys. C: Solid State Phys., 1986, 19, p. 5689-5694.

20.    Verma A. S., Bhardwaj S. R., Mechanical and optical properties of A^IIB^IVC₂^V and A^IB^IIIC₂^VI semiconductors, Phys. Stat. Solidi (b), 2006, 243, p. 2858-2863.

21.    Kumar V., Srivastava A.K., Jha V., Bulk modulus and microhardness of tetrahedral semiconductors, Jour. Phys. Chem. Solids, 2010, 71, p. 1513-1520.

22.    Gorai S. K., Mahto P., Plasmon energy, bulk modulus, electronic polarisability of A^IB^IIIC₂^VI and A^IIB^IVC₂^V ternary chalcopyrite semiconductors, Indian Jour. Phys. 2010, 84, p. 587-592.

23.    Phillips J. C., Bonds and Bands in Semiconductors, New York, Academic Press, 1973.

24.    Ashcroft N.W., Mermin N. D., Solid State Physics, Saunders, 1976.

25.    Neumann H., Trends in the thermal expansion coefficients of the A^IB^IIIC₂^VI and A^IIB^IVC₂^V chalcopyrite compounds, Kristall und Technik., 1980, 15, p. 849-957.

26.    Garbato L., Rucci A., Microhardness of ternary chalcopyrite semiconductors, Phil. Mag., 1977, 35, p. 1685-1688.

27.    Lide D.R. (Ed.), CRC Handbook of Chem. and Phys., 80^th, CRC Press, 1999.