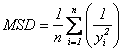Taguchi Optimization of Process Parameters on the Hardness and Impact Energy of Aluminium Alloy Sand Castings

John O. OJI¹*, Pamtoks H. SUNDAY², Omolayo M. PETINRIN¹, Adelana R. ADETUNJI^1,3

¹ Prototype Engineering Development Institute Ilesa, Osun State. Nigeria.

²Nigerian National Petroleum Corporation, Abuja. Nigeria.

³Department of Materials Science & Engineering, Obafemi Awolowo University, Ile-Ife, Nigeria.

E-mail: ojiortega@gmail.com; pamtoks4u@gmail.com; layopet01@yahoo.com; aderade2004@yahoo.com

* Corresponding author: Phone: +234(0)7084089009

Abstract

An optimization technique for sand casting process parameters based on the Taguchi method is reported in this paper. While keeping other casting parameters constant, aluminium alloy castings were prepared by sand casting technique using three different parameters, namely the mould temperature, pouring temperature and runner size. Hardness and impact energy tests were done for the resulted castings. The settings of parameters were determined by using the Taguchi experimental design method. The level of importance of the parameters on the hardness impact energy was determined using the analysis of variance (ANOVA). The optimum parameter combination was obtained by using the analysis of signal-to-noise (S/N) ratio. Analysis of the results shows that 100°C mould temperature and 700°C pouring temperatures are optimal values for hardness and impact energy. However 200 mm² and 285 mm² runner sizes are the optimal values for hardness and impact energy respectively. The mould temperature was the most influential parameter on the hardness impact energy of the castings.

Keywords

Taguchi method; Optimization; Sand casting; Aluminium alloy; Process parameters; Hardness; Impact energy; ANOVA.

Introduction

The wide range of the application of aluminium alloys is very obvious. Their desirable characteristics of light weight, excellent resistance to corrosion in the atmosphere and water, strength [1] and high thermal conductivity gives them an edge over other metals in the electrical, aviation, marine, aerospace, construction and automotive industries just to mention but a few [2]. This increased usage creates the need for a deeper understanding of their mechanical behaviour and the influences of processing parameters, [3, 4]. This knowledge enables the designer to ensure that the casting will achieve the desired properties for its intended application [5, 6].

There is no doubt that casting as a process involves so many parameters [7-9]such as melting temperature of the charge, temperature of the mould, pouring speed, pouring temperature, composition, microstructure, size of casting, runner size, composition of the alloy and solidification time just to mention but a few. [7-12] just to mention but a few have successfully carried out studies on the varying effects of casting process parameters on the mechanical properties of casted metals and their alloys.

One of the recent most important optimization processes is the Taguchi method [13] conceived and developed by Japanese scholar Engr. Dr. Genichi Taguchi in 1950. Taguchi technique is a powerful tool for the design of high quality systems [14, 15]. It provides a simple efficient and systematic approach to optimize design for performance, quality and cost. The methodology is valuable when design parameters are qualitative and discrete. Taguchi parameter design can optimize the performance characteristic through the setting of design parameters and reduce the sensitivity of the system performance to source of variation [16, 17]. The Taguchi approach enables a comprehensive understanding of the individual and combined from a minimum number of simulation trials. This technique is multi – step process which follow a certain sequence for the experiments to yield an improved understanding of product or process performance [18].

The objective of this study is to determine the optimal settings of sand casting process parameters using Taguchi’s experimental design method. Orthogonal arrays of Taguchi, the signal-to-noise (S/N) ratio, the analysis of variance (ANOVA), and regression analyses are employed to find the optimal levels and to analyze the effect of the casting process parameters on hardness and impact energy values.

Experimental Design

The steps applied for Taguchi optimization in this study are as follows.

Ø Select noise and control factors (process parameters in this case)

Ø Select Taguchi orthogonal array

Ø Conduct Experiments

Ø Hardness and Impact Energy measurements

Ø Analyze results; (Signal to noise ratio)

Ø Predict optimum performance

Ø Confirmation experiment

The standard Taguchi L₉ Orthogonal Array (OA) format is chosen from preliminary works [10, 19, 20] by the authors which identified three parameters namely the mould temperature, pouring temperature and runner size as important casting process variables which affect the mechanical properties. Sufficient details of the effect of different parameter values on experimental results can be obtained by choosing three levels for each parameter to investigate. The criteria used for choosing the three parameter levels are to explore a maximum range of experimental variables. In addition, it is unnecessary to have uniformly spaced levels because of the counterbalance property of the Orthogonal Array [21]. Previous work by [11] has shown that the optimum pouring temperature range is 700-750°C so for this study the temperature level range is 700-750°C. The three levels for mould temperature parameter and runner size parameter are selected also according to literature reviews and previous casting experiences.

Taguchi methods which combine the experiment design theory and the quality loss function concept have been used in developing robust designs of products and processes and in solving some taxing problems of manufacturing/production [22]. The degrees of freedom for three parameters in each of three levels were calculated as follows [14].

Degree of Freedom (DOF) = number of levels -1. For each factor, DOF equal to: For (Mould temperature); DOF = 3 – 1 = 2; For (Pouring Temperature); DOF = 3 – 1 = 2; For (Runner size); DOF = 3 – 1 = 2

In this study nine experiments were conducted at different parameters. For this Taguchi L₉ orthogonal array was used, which has nine rows corresponding to the number of tests, with three columns at three levels. L₉ OA has eight DOF, in which 6 were assigned to three factors (each one 2 DOF) and 2 DOF was assigned to the error. For the purpose of observing the degree of influence of the process parameters, three factors, each at three levels, are taken into account, as shown in Tables 2.

Analysis of the S/N Ratio

Taguchi method uses the (signal – to – noise (S/N) ratio, because it minimizes quality characteristic variation due to uncontrollable parameter. The hardness and impact energy is the objective function so that "the larger-the-better" S/N ratio is chosen. The S/N ratio used for this type of response is given by [14]. The S/N ratio for the larger-the-better is:

S/NLTB = -10log[MSD]	(1)
	(2)

where: n is the number of measurements in a trial/row, in this case, n=1 and y is the measured value in a run/row. The S/N ratio values are calculated by taking into consideration equation. 1. The S/N ratio values obtained for this experiment are as shown in table 6 and 7 respectively.

Analysis of variance (ANOVA)

Analysis of Variance (ANOVA) is a computational technique to quantitatively estimate the relative contribution, which each controlled parameter makes to the overall measured response and expressing it as a percentage. ANOVA uses the S/N ratio responses for these calculations. The basic idea of ANOVA is that the total sum of squares of the standard deviation is equal to the sum of squares of the standard deviation caused by each parameter. The total sum of squared deviations SS_T from the total mean S/N ratio η_m can be calculated as [23]:

(3)

where n is the number of experiments in the orthogonal array and η_i is the mean S/N ratio for the i^th experiment.

The percentage contribution, P of the process parameters on the hardness and impact energy (shown as table 8 and 9) can be calculated as:

(4)

Results and Discussions

Table 1 shows the chemical composition of the aluminium alloy employed in the foundry process for this analysis.

Table 1. Chemical composition of aluminium alloy

Element	Al	Fe	Si
Weight Percentage (W %)	97.2	0.7	2.1
Concentration (mgl)	972	7	21

A standard Taguchi L₉ Orthogonal Array (OA) is chosen for this study as it can operate three parameters, each at three levels and presented in Table 2 and 3.

Table 2. Process parameters and their values at 3 levels

Process Parameters	LEVELS
	L1	L2	L3
Mould Tempt (°C)	100	150	170
Pouring Tempt (°C)	700	720	750
Runner Size (mm^2)	180	200	285

Table 3.Standard L₉ array for hardness and impact energy

Expt No	Mould Tempt (°C)	Pouring Tempt (°C)	Runner Size (mm^2)	Hardness (HRB)	Impact Energy (Joule)
1	100	700	180	15.08	46
2	100	720	200	18.22	31
3	100	750	285	16.47	44
4	150	700	200	17.60	32
5	150	720	285	17.40	21
6	150	750	180	14.00	25
7	170	700	285	12.10	18
8	170	720	180	10.02	12
9	170	750	200	11.42	14

The hardness and impact energy values measured from the experiments and their corresponding S/N ratio values are listed in Table 4 and 5.

Table 4. Hardness values and S/N ratio values for experiments

Expt. no	Hardness (HRB)	S/N Ratio (dB)
1	15.08	23.57
2	18.22	25.21
3	16.47	24.33
4	17.60	24.91
5	17.40	24.81
6	14.00	22.92
7	12.10	21.66
8	10.02	20.02
9	11.42	21.15

Table 5. Impact energy values and S/N ratio values for experiments

Expt No	Impact Energy (Joule)	S/N Ratio (dB)
1	46	33.26
2	31	29.83
3	44	32.87
4	32	30.10
5	21	26.44
6	25	27.96
7	18	25.10
8	12	21.58
9	14	22.92

The response table by factor level for the mould temperature, pouring temperature and runner size was created in the integrated manner and the results are given in Table 6 and 7.

Table 6. S/N ratio values for hardness by factor level

LEVEL	Mould Tempt (°C)	Pouring Tempt  (°C)	Runner Size  (mm^2)
1	24.37*	23.38*	21.17
2	24.21	23.35	23.76*
3	20.94	22.80	23.60
Delta	3.43	0.58	2.59
Rank	1	3	2

*Optimum Level

Table 7. S/N ratio values for impact energy by factor level

LEVEL	Mould Tempt  (°C)	Pouring Tempt (°C)	Runner Size (mm^2)
1	31.99*	29.49*	27.60
2	28.17	25.95	27.68
3	23.20	27.92	28.14*
Delta	8.79	3.54	0.54
Rank	1	2	3

*Optimum Level

Statistically, an F test, named after Fisher [16] is used to determine design parameters which have a significant effect on the quality characteristic. In the analysis, the F-ratio is a ratio of the mean square error to the residual error, and is traditionally used to determine the significance of a factor. Percent (%) is defined as the significance rate of the process parameters on the hardness and impact energy. The percent numbers depict that the mould temperature, pouring temperature and runner size have significant effects on the hardness and impact energy values. It can also be observed from Table 8 that within the selected experimental design, the pouring temperature, mould temperature, and runner size affect the hardness by 2.27%, 77.46% and 16.55% respectively.

Table 8. ANOVA results for hardness of aluminium alloy castings

Source of Variation	Degree of freedom (DOF)	Sum of squares (SS)	Variance (V)	F-ratio (F)	Percentage Contribution (P)
Mould Tempt (°C)	2	21.48	10.74	20.85	77.46%
Pouring Tempt (°C)	2	0.63	0.31	0.61	2.27%
Runner Size (mm^2)	2	4.59	2.29	4.46	16.55%
Error	2	1.03	0.51		3.72%
Total	8	27.73			100%

Table 9 shows also that within the selected experimental design, the pouring temperature, moulding temperature, and runner size affect the impact energy by 13.32%, 86.12% and 0.38% respectively.

Table 9. ANOVA results for impact energy of aluminium alloy castings.

Source of Variation	Degree of freedom (DOF)	Sum of squares (SS)	Variance (V)	F-ratio (F)	Percentage Contribution (P)
Mould Tempt (°C)	2	115.55	57.76	444.42	86.12%
Pouring Tempt (°C)	2	17.87	8.94	68.73	13.32%
Runner Size (mm^2)	2	0.51	0.26	1.96	0.38%
Error	2	0.26	0.13		0.18%
Total	8	134.19			100%

Regardless of the category of the performance characteristics, a greater S/N value corresponds to a better performance. Therefore, the optimal level of the casting process parameters is the level with the greatest S/N value. Based on the analysis of the S/N ratio, the optimal hardness was obtained at 100°C mould temperature (level 1), 700°C pouring temperature (level 1) and 200mm² runner size (level 3). The varying effects of process parameters on the hardness values are shown in figure 1a, 1b, and 1c.

The hardness decreases with increase in mould temperature (above 100°C) and pouring temperature (above 700°C). An increase in runner size however produced increase in the hardness. Also, the optimal impact energy was obtained at 100°C mould temperature (level 1), 700°C pouring temperature (level 1) and 285mm² runner size (level 3). The varying effects of the processing parameters on the impact energy values are shown in figure 2a, 2b, and 2c. The hardness decreases with increase in mould temperature (above 100°C) and pouring temperature (above 700°C but begins to rise on reaching 720°C). However an increase in runner size produced increase in the impact energy.

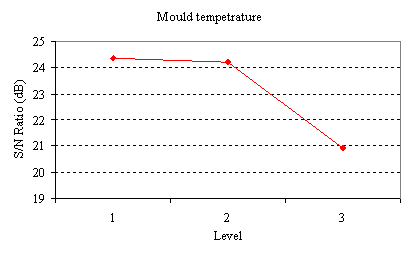
Figure 1a. Main effects plot for mould temperature S/N ratio values on hardness

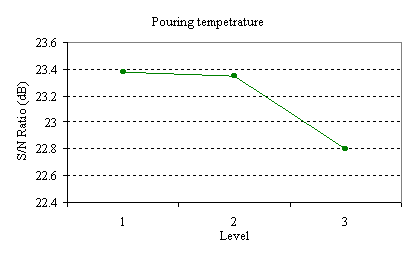
Figure 1b. Main effects plot for pouring temperature S/N ratio values on hardness

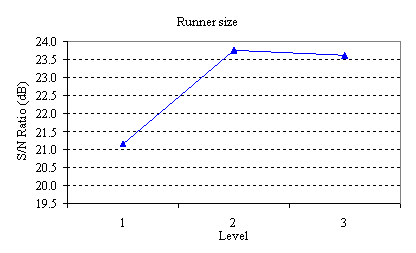
Figure 1c. Main effects plot for runner size S/N ratio values on hardness

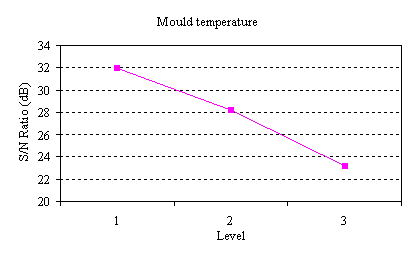
Figure 2a. Main effects plot for mould temperature S/N ratio values on impact energy

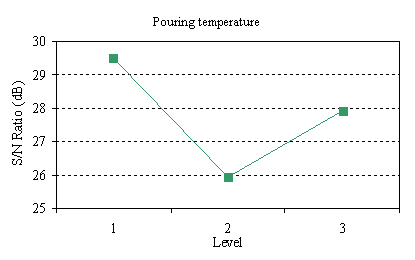
Figure 2b. Main effects plot for pouring temperature S/N ratio values on impact energy

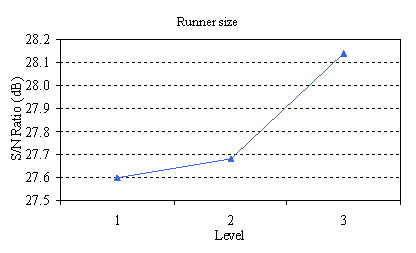
Figure 2c. Main effects plot for runner size S/N ratio values on impact energy

Conclusions

This study has discussed an application of the Taguchi method for investigating the effects of sand casting process parameters on the hardness and impact energy values of aluminium alloy castings. From the analysis of the results using the conceptual signal-to-noise (S/N) ratio approach, regression analysis, analysis of variance (ANOVA), and Taguchi’s optimization method, the following can be concluded from the present study:

· A statistically designed experiment based on Taguchi method was performed using L₉ orthogonal array to analyze the hardness and impact energy as response variables.

· Within the experimental level ranges, the most significant influencing parameter on hardness is the mould temperature, which accounts for 77.46% of the total effect, followed by the runner size (16.55%), and mould temperature (2.27%) respectively. On the other hand the most significant influencing parameter on the impact energy is the mould temperature, which accounts for 86.12% of the total effect, followed by the pouring temperature (13.32%), and runner size (0.38%) respectively.

Acknowledgement

The authors are grateful to Engr S.I. Yakubu of the National Metallurgical Development Centre (NMDC) Jos, Nigeria for the synthesis of this work.

References

1.  Allen D. K., Metallurgy Theory and Practice. American Technical Society, Chicago (ASME), 1979, p 238-239.

2. Karl B., “Metal Casting”, Quickest and Least Expensive Route to a Near Shape Product. Reference Book for MY4130. Materials Science and Engineering Department, Michigan Technical University, USA, 2005, p 150-165.

3. Mrwَka-N. G., Sieniawski J., Wierzbi S. M., Analysis of intermetalic particles In AlSi1MgMn aluminium alloy. Journal of Achievements in Materials and Manufacturing Engineering, 2007, 20, p. 155-158.

4. Kauffman J.G., Rooy E. L., Aluminium Alloy Castings. ASM International, Ohio, 2005.

5. Li Z., Samuel A.M., Samuel F. H., Ravindran C., Valtierra S., Doty H. W., Parameters controlling the performance of AA319-type alloys Part I. Tensile properties. Elsevier Journal of Materials Science and Engineering, 2004, 367, p. 96-110.

6. Shabestari S. G., Moemeni, H., Effect of copper and solidification conditions on the microstructure and mechanical properties of Al–Si–Mg alloys. Journal of Materials Processing Technology, 2004, p. 153-154, 193-198.

7. El-Aini H A.., Hassan M. K., Mohammed Y., Effect of mould types and cooling rate on mechanical properties of Al alloy 6061 within ceramic additives. The 2nd International Conference on Energy Engineering ICEE-2, December 27-29, 2010 - Aswan, Egypt.

8. Timelli G., Bonollo F., The influence of Cr content on the microstructure and mechanical properties of AlSi₉Cu₃(Fe) die casting alloys. Elsevier Journal of Material Science and Engineering, 2010, A, 528, p. 273-282.

9. Lee C. D., Effects of microporosity on tensile properties of A356 aluminium alloy. Elsevier Journal of Material Science and Engineering, 2007, A, 464, p. 249-254.

10. Oji J. O., Kareem B., Idusuyi N., Effects of Mould and Pouring Temperatures on the Ultimate Tensile Strength of Aluminium Alloy Sand Castings: An ANOVA Approach. LEJPT, Academic Direct, 2011, 19(10), p. 97-108.

11. Mohammad B. N., Akpan, P. P., Behaviour of Aluminium Alloy Castings under Different Pouring Temperatures and Speeds. LEJPT, Academic Direct, 2007, 11, p. 71-80.

12. Boileau J. M., Zindel J. W., Allison J. E., The effect of solidification time on the mechanical properties in a cast A356- T6 aluminium alloy. Society of Automotive Engineers, Inc, 1997.

13. Hu H., Squeeze casting of magnesium alloys and their composite, Elsevier Journal of Material Science, 1998, 33, p. 1579-1589.

14. Taguchi G., Hocheng H., Taguchi methods, orthogonal arrays and linear graphs, tools for quality engineering, Dearborn, MI: American Supplier Institute, 1987, p. 35-38.

15. Taguchi G., Taguchi on robust technology development methods. New York, NY: ASME press, 1993, p. 1-40.

16. Ross P. J., Taguchi technique for quality engineering. McGRAW-Hill International Editions, Singapore, 1996.

17. Roy R. K., A primer on Taguchi method. New York: Van Nostrad Reinhold, 1990.

18. Basavarajappa S., Chandramohan G., Paulo D. J., Application of Taguchi techniques to study dry sliding wear behaviour of metal matrix composites, Materials and Design, 2007, 28, p. 1393-1398.

19. Oji J. O., Pamtoks S. H., Optimizing the effect of sand casting process parameters on the mechanical properties of an aluminium alloy part. Unpublished HND Project Submitted to the Department of Mechanical Engineering, The Federal Polytechnic Bauchi, 2007.

20. Oji J. O., Pamtoks S. H., Idusuyi N., Aliu T. O., Statistical Model for Predicting the Hardness of Aluminium Alloy Sand Castings under Different Process Parameters.New York Science Journal, 2012, 5(10), p. 20-24.

21. Fowlkes W. Y., Creveling C. M., Engineering methods for robust product design, Using Taguchi Methods in technology and product development. (Addison-Wesley Publishing Company), 1995.

22. Tsao C. C., Hocheng H., Taguchi analysis of delamination associated with various drill bits in drilling of composite material. International Journal of Machine Tools Manufacturing, 2004, 44, p. 1085-1090.

23. Lindman H., Analysis of variance in experimental design. (Springer-Verlag), New York, 1992.